Today you will…
Learn about line symmetry and rotational symmetry and how they are related.
Learners of mathematics are often asked to state the number of lines of symmetry and the order of rotational symmetry of given two-dimensional shapes. This lesson turns that around and asks KS3 learners to invent shapes with particular symmetries. Constructing their own drawings gives learners the opportunity to be creative and independent, and helps them to see what features of shapes cause them to have line or rotational symmetry.
Starter activity
Project (or copy) these symbols (or similar ones) onto the board:
Q: Which of these shapes and symbols do you recognise? Where have you seen them before?
Learners will be familiar with many of the symbols and will know what some of them refer to. They might give names for some of them, such as pentagram for the five-pointed star, although technically a pentagram shows the lines inside (star outline), and (solid star) would be better described as a concave decagon. In the discussion, some cross-curricular links could be made (e.g., with music and the ‘sharp’ sign).
Q: Are any of these shapes symmetrical?
Learners might interpret ‘symmetrical’ in different ways. They could come to the board so that they can point to features of the shapes to explain their meaning. (Or, harder, they could try to describe what they mean in words only.) They are likely to talk about turning the shapes over (reversing them: line or mirror symmetry) and turning them around (rotating them: rotational symmetry). In the discussion, you could draw attention to the terms line of symmetry, centre of rotation and order of rotational symmetry.
Q: How many lines of symmetry do these shapes have? What are their orders of rotational symmetry?
Learners will need to look carefully at some of the shapes; e.g., the scissors don’t have a line of symmetry, but at first sight they might appear to! Learners might disagree about some of the answers, depending on how equal some of the features look in some of the drawings. This is a common problem with ‘real-life objects’ – you could pick up on this by asking whether a human face is symmetrical. (Fashion models sometimes have very symmetrical faces, but most of us don’t.)
The answers are:

Main Activities
1: Shading four squares
Give learners a sheet of 1 cm x 1 cm squared paper and ask them to draw a 3 by 3 square. (Alternatively, you could give them a sheet with lots of 3 by 3 squares already drawn for them.)
Q: You’ve got 9 little squares in the big square. You’re going to make a design by shading exactly 4 of those 9 squares, but they can be whichever 4 you like. Can you shade 4 squares so that the finished drawing has 4 lines of symmetry?
There are 2 ways of doing it
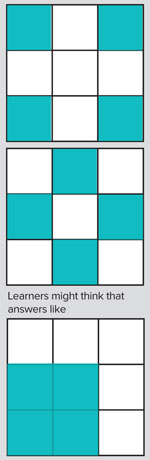
Learners might think that answers like also count, which they do if you just look at the shaded squares, but not if you consider the drawing as a whole. Learners could show their ideas on the board and someone else could draw in the lines of symmetry (perhaps as dashed lines).
Q: What other numbers of lines of symmetry can you produce? What different orders of rotational symmetry? Try to make as many different possible answers as you can – but only shading four squares each time!
Learners could work for a while on this. As they go, you could throw out additional challenges, such as:
Q: Can you do one that has a number of lines of symmetry that is different from the order of rotational symmetry?
Q: Can you make the order of rotational symmetry more than the number of lines of symmetry?
Q: Can you do it the other way round?
2: Shading five squares
Q: Suppose you are allowed an extra shaded square. Does that make it easier or harder?
Give learners time to explore this related problem. They may be surprised to discover that in essence it is exactly the same problem as the four-squares task. This is because choosing 4 of the 9 squares to shade is equivalent to choosing not to shade the other 5. So the answers will be the ‘negatives’ of the answers to the four-squares task.
3: Other shadings
Learners could work on shading just 3 squares, or just 2 or just 1. What possible orders of rotational symmetry and numbers of lines of symmetry can they produce? Confident learners could try to put all their results together and summarise.
Home learning
What happens if you are allowed two different colours for your shaded squares? Colour affects symmetry. For example, if you have to shade two squares red and two squares blue, what possible orders of rotational symmetry and numbers of lines of symmetry can you produce?
Summary
You could conclude the lesson with a plenary in which learners share their creations and show the different symmetries that they were able to construct. Some learners may have discovered ways of creating rotational symmetry without any lines of symmetry. Did anyone manage the opposite?
Info bar
Stretch them further
Learners who are comfortable with the main task in this lesson could try it on isometric paper instead, drawing a 3 by 3 by 3 equilateral triangle containing 9 small equilateral triangles. If they are allowed to shade exactly 4 of the small triangles, what possible numbers of lines of symmetry and orders of rotational symmetry can they create this time?
Additional resources
A very nice resource, particularly for use on an electronic whiteboard, is at tinyurl.com/29sw8c, where learners can make their drawings while seeing various symmetrical versions (reflections, rotations, etc.) appearing in real time. This can be a good stimulus for discussion if learners are asked to predict what will happen under particular circumstances.
About the expert
Colin Foster is a Senior Research Fellow in the School of Education at the University of Nottingham, and writes books for mathematics teachers (www.foster77.co.uk).
For a huge selection of free maths lesson plans for KS3 and KS4 click here.









